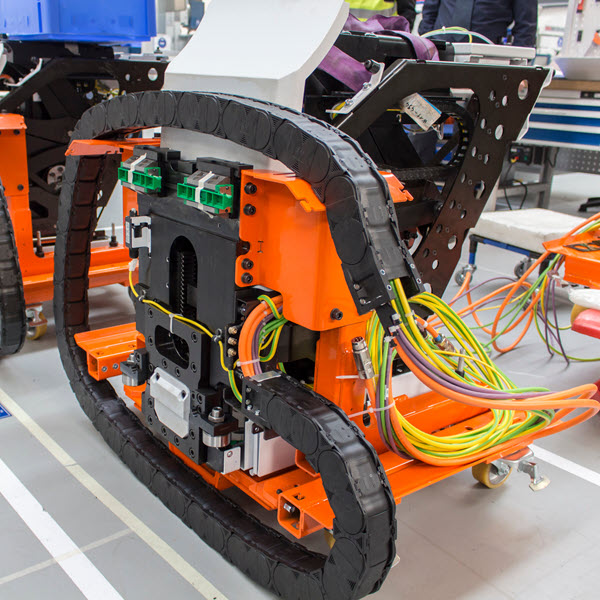
How do you calculate Surface Pressure for bearings and why is it so important?
Understanding how to calculate the surface pressure will help when deciding which plain bearing is the most ideal for your application. Although this is not essential, an estimate with a large safety factor would be a good guess but knowing the surface pressure is one factor that helps determine which bearing would be optimal. So, what is surface pressure, why is important and how does it work in helping identify the best plain bearing?
Not sure what bearing you need? Read our blogs on igus® plain bearings to help you decide here.
The what, the why and the how
What is surface pressure? The term refers to the force acting on a specific area of the surface. The easiest way to understand is to use an example:

If a bearing needs to be able to withstand 1 ton you need to determine how much surface area the load will be spread over? Think about an elephant’s foot and a stiletto heel on a shoe. The elephant’s foot has a large area to distribute the weight on, but the stiletto has a very small surface area where the heel meets the ground. The elephant has distributed the weight across the whole surface which means it doesn’t sink deep into the ground, but the lady in the stiletto doesn’t have the same surface for distributing the weight so sinks, thus demonstrating that the surface pressure on the stiletto is higher even though the elephant clearly has a larger force through its foot. The elephant can support the ton weight, the stiletto cannot. The situation is similar with plain bearings.
There is a simple equation that we can use to accurately calculate the projected surface area and therefore demonstrate the resulting pressure that the plain bearing will absorb. Surface pressure calculation determines force within an area for an individual unit so in numerical terms, Force (F) divided by Area (A) equals Surface Pressure (P) P= F/A
Calculating surface pressure is a simple and straightforward proposition if you have the correct numerical factors for carrying out the equation. The load is typically a direct measurement in kg however this needs to be converted to the force (F) in Newtons (N)
To determine the projected surface area in the case of igus® plain bearings (A) simply multiply the overall length by its inside diameter giving a projected surface area in mm²Now divide Force by Area squared to equal the total Surface Pressure. We use the projected surface area to simplify and automatically allow for a safety factor knowing that in reality the area is larger.
When calculating the surface pressure for a plain bearing, these calculations enable you to get the results of the inner cross-section of the plain bearing and the simplified load share (projected area). It is important to remember that not every section within the bearing carries the same load. The simple calculation that uses the bearing length and diameter is ultimately the more practical and precise one. With polymer plain bearings, it is especially important that the load is distributed over as large of an area as possible, since its compressive strength is lower than that of steel.
The above calculation allows us to know half of what we need to calculate the surface pressure. Now we need to denote the “N” in the surface pressure unit which is N/mm² also often referred to as ‘megapascals’ (MPa)
As mentioned previously the N stands for Newton and in order to establish an accurate calculation, we need to multiply the load that a bearing distributes by a factor of 9.81 (this is the conversion factor from kg to N), but most people multiply by 10 to keep it simple. Here is an example of the calculation:
Force (N) = load (kg) x 9.81
Calculate surface pressure: P=F/A
The force is distributed over the entire bearing surface. All that’s left to do is to divide the force by the calculated area to get the surface pressure.
There are still numerous parameters to consider when ensuring the bearing will be the right one for your application, but this is a good place to start!